The Gravimagnetic Parameter ``Ecology'' of Magnetic Rotators Nomenclature

A Universal Diagram for Gravimagnetic Rotators

  

The classification  given above was based on relations between the characteristic radii, i.e. quantities which cannot be observed directly. This drawback can be removed if we note that the light cylinder radius , Schwartzman radius  and corotation radius  are functions of the well-observed quantity, rotational period of the magnetor  p. Hence, the above classification can be reformulated in the form of inequalities for the rotational period of a magnetic rotator.

One can introduce two critical periods and such that their relationship with period p of a magnetic rotator specifies the rotator's type:

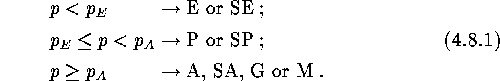
The values of and can be determined from Table 2 which defines the basic nomenclature, and are functions of the parameters , , and . The parameters p and characterize the electromagnetic interaction, while describes the gravitational interaction. Instead of , we introduce the potential accretion luminosity L

The physical sense of the potential luminosity is quite clear: the accreting star would be observed to have this luminosity if the matter formally falling on the gravitational capture cross-section were to reach its surface.

Approximate expressions for critical periods (Lipunov 1992[107]) are

 

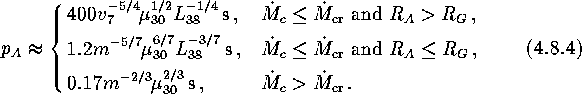
Here a new critical period was introduced from the condition :

Treating the rotator's magnetic dipole moment and as parameters, we find that an overwhelming majority of the magnetor's stages can be shown on a `` '' diagram (Lipunov 1982a[98]). The quantity L also proves to be convenient because it can be observed directly at the accretion stage.